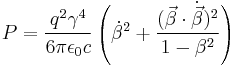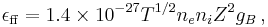Bremsstrahlung
Bremsstrahlung (German pronunciation: [ˈbʁɛmsˌʃtʁaːlʊŋ] (![]() listen), from bremsen "to brake" and Strahlung "radiation", i.e. "braking radiation" or "deceleration radiation"), is electromagnetic radiation produced by the acceleration of a charged particle, such as an electron, when deflected by another charged particle, such as an atomic nucleus. The term is also used to refer to the process of producing the radiation. Bremsstrahlung has a continuous spectrum, which becomes more intense and shifts toward higher frequencies when the energy of the accelerated particles is increased. The phenomenon was discovered by Nikola Tesla[1] during high frequency research he conducted between 1888 and 1897.
listen), from bremsen "to brake" and Strahlung "radiation", i.e. "braking radiation" or "deceleration radiation"), is electromagnetic radiation produced by the acceleration of a charged particle, such as an electron, when deflected by another charged particle, such as an atomic nucleus. The term is also used to refer to the process of producing the radiation. Bremsstrahlung has a continuous spectrum, which becomes more intense and shifts toward higher frequencies when the energy of the accelerated particles is increased. The phenomenon was discovered by Nikola Tesla[1] during high frequency research he conducted between 1888 and 1897.
Strictly speaking, bremsstrahlung refers to any radiation due to the acceleration of a charged particle, which includes synchrotron radiation; however, it is frequently used in the more narrow sense of radiation from electrons stopping in matter.
Bremsstrahlung emitted from plasma is sometimes referred to as free-free radiation. This refers to the fact that the radiation in this case is created by charged particles that are free both before and after the deflection (acceleration) that causes the emission.
Contents |
Dipole approximation
Suppose that a particle of charge 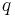 experiences an acceleration
experiences an acceleration 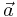 which is collinear with its velocity
which is collinear with its velocity 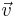 (this is the relevant case for linear accelerators). Then, the relativistic expression for the angular distribution of the bremsstrahlung (considering only the dominant dipole radiation contribution), is
(this is the relevant case for linear accelerators). Then, the relativistic expression for the angular distribution of the bremsstrahlung (considering only the dominant dipole radiation contribution), is
-
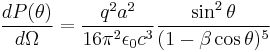 ,
,
- where
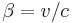 and
and 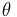 is the angle between
is the angle between 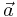 and the point of observation.
and the point of observation.
Integrating over all angles then gives the total power emitted as [2]
-
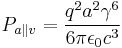 ,
,
where  is the Lorentz factor .
is the Lorentz factor .
The general expression for the total radiated power is[3]
where 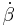 signifies a time derivative of
signifies a time derivative of 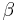 . Note, this general expression for total radiated power simplifies to the above expression for the specific case of acceleration parallel to velocity (
. Note, this general expression for total radiated power simplifies to the above expression for the specific case of acceleration parallel to velocity (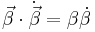 ), by noting that
), by noting that 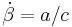 and
and  . For the case of acceleration perpendicular to the velocity (
. For the case of acceleration perpendicular to the velocity (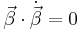 ) (a case that arises in circular particle accelerators known as synchrotrons), the total power radiated reduces to
) (a case that arises in circular particle accelerators known as synchrotrons), the total power radiated reduces to
-
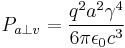 .
.
The total power radiated in the two limiting cases is proportional to  (
( ) or
) or  (
( ). Since
). Since 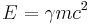 , we see that the total radiated power goes as
, we see that the total radiated power goes as  or
or 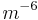 , which accounts for why electrons lose energy to bremsstrahlung radiation much more rapidly than heavier charged particles (e.g., muons, protons, alpha particles). This is the reason a TeV energy electron-positron collider (such as the proposed International Linear Collider) cannot use a circular tunnel (requiring constant acceleration), while a proton-proton collider (such as the Large Hadron Collider) can utilize a circular tunnel. The electrons lose energy due to bremsstrahlung at a rate
, which accounts for why electrons lose energy to bremsstrahlung radiation much more rapidly than heavier charged particles (e.g., muons, protons, alpha particles). This is the reason a TeV energy electron-positron collider (such as the proposed International Linear Collider) cannot use a circular tunnel (requiring constant acceleration), while a proton-proton collider (such as the Large Hadron Collider) can utilize a circular tunnel. The electrons lose energy due to bremsstrahlung at a rate  times higher than protons do.
times higher than protons do.
Thermal Bremsstrahlung
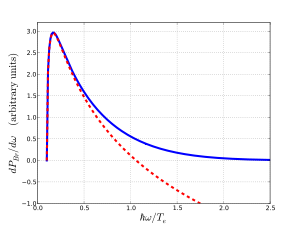
 , and is also suppressed near
, and is also suppressed near 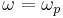 . This plot is for the quantum case
. This plot is for the quantum case 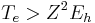 , and
, and 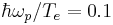 .
.In a plasma the free electrons are constantly producing Bremsstrahlung in collisions with the ions. A complete analysis requires accounting for both binary Coulomb collisions as well as collective (dielectric) behavior. A detailed treatment is given in [4], some of which is summarized in [?], while a simplified one is given in [5]. In this section we follow Bekefi's dielectric treatment, with collisions included approximately via the cutoff wavenumber 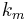 .
.
Consider a uniform plasma, with thermal electrons (distributed according to the Maxwell–Boltzmann distribution with the temperature 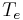 ). Following Bekefi, the power spectral density (power per angular frequency interval per volume, integrated over the whole
). Following Bekefi, the power spectral density (power per angular frequency interval per volume, integrated over the whole 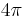 sr of solid angle, and in both polarizations) of the Bremsstrahlung radiated, is calculated to be
sr of solid angle, and in both polarizations) of the Bremsstrahlung radiated, is calculated to be
![{dP_\mathrm{Br} \over d\omega} = {8\sqrt 2 \over 3\sqrt\pi} \left[1-{\omega_p^2 \over \omega^2}\right]^{1/2} \left[ Z_i^2 n_i n_e r_e^3 \right]
\left[ { \frac{(m_ec^2)^{3/2}}{(k_B T_e)^{1/2}} } \right] E_1(y) ,](/2010-wikipedia_en_wp1-0.8_orig_2010-12/I/a3a85fca54a66dc22aaacbbb2020c681.png)
where 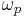 is the electron plasma frequency,
is the electron plasma frequency, 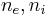 is the number density of electrons and ions,
is the number density of electrons and ions,  is the classical radius of electron,
is the classical radius of electron,  is its mass,
is its mass, 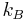 is the Boltzmann constant, and
is the Boltzmann constant, and  is the speed of light. The first bracketed factor is the index of refraction of a light wave in a plasma, and shows that emission is greatly suppressed for
is the speed of light. The first bracketed factor is the index of refraction of a light wave in a plasma, and shows that emission is greatly suppressed for  (this is the cutoff condition for a light wave in a plasma; in this case the light wave is evanescent). This formula thus only applies for
(this is the cutoff condition for a light wave in a plasma; in this case the light wave is evanescent). This formula thus only applies for  . Note that the second bracketed factor has units of 1/volume and the third factor has units of energy, giving the correct total units of energy/volume. This formula should be summed over ion species in a multi-species plasma.
. Note that the second bracketed factor has units of 1/volume and the third factor has units of energy, giving the correct total units of energy/volume. This formula should be summed over ion species in a multi-species plasma.
The special function 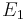 is defined in the exponential integral article, and the unitless quantity
is defined in the exponential integral article, and the unitless quantity 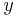 is
is
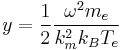
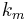 is a maximum or cutoff wavenumber, arising due to binary collisions, and can vary with ion species. Roughly,
is a maximum or cutoff wavenumber, arising due to binary collisions, and can vary with ion species. Roughly, 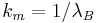 when
when 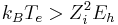 (typical in plasmas that are not too cold), where
(typical in plasmas that are not too cold), where 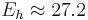 eV is the Hartree energy, and
eV is the Hartree energy, and  is the electron thermal de Broglie wavelength. Otherwise,
is the electron thermal de Broglie wavelength. Otherwise,  where
where 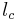 is the classical Coulomb distance of closest approach.
is the classical Coulomb distance of closest approach.
For the usual case 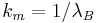 , we find
, we find
![y = {1\over2}\left[\frac{\hbar\omega}{k_B T_e}\right]^2](/2010-wikipedia_en_wp1-0.8_orig_2010-12/I/3b3b3a2be22b09871744e7a805e3d276.png) .
.
The formula for 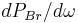 is approximate, in that it neglects enhanced emission occurring for
is approximate, in that it neglects enhanced emission occurring for  slightly above
slightly above 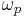 .
.
In the limit 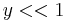 , we can approximate E1 as
, we can approximate E1 as ![E_1(y) \approx -\ln [y e^\gamma] + O(y)](/2010-wikipedia_en_wp1-0.8_orig_2010-12/I/1887614c3a8231e4f542fa437af642af.png) where
where  is the Euler-Mascheroni constant. The leading, logarithmic term is frequently used, and resembles the Coulomb logarithm that occurs in other collisional plasma calculations. For
is the Euler-Mascheroni constant. The leading, logarithmic term is frequently used, and resembles the Coulomb logarithm that occurs in other collisional plasma calculations. For 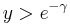 the log term is negative, and the approximation is clearly inadequate. Bekefi gives corrected expressions for the logarthmic term that match detailed binary-collision calculations.
the log term is negative, and the approximation is clearly inadequate. Bekefi gives corrected expressions for the logarthmic term that match detailed binary-collision calculations.
The total emission power density, integrated over all frequencies, is
![\begin{align}
P_\mathrm{Br} &= \int_{\omega_p}^\infty d\omega {dP_\mathrm{Br}\over d\omega} = {16 \over 3} \left[ Z_i^2 n_i n_e r_e^3 \right]
\left[m_e c^3 \right] k_m G(y_p) \\
G(y_p) &= {1 \over 2\sqrt{\pi}} \int_{y_p}^\infty dy y^{-1/2} \left[1-{y_p\over y}\right]^{1/2} E_1(y) \\
y_p &= y(\omega=\omega_p)
\end{align}](/2010-wikipedia_en_wp1-0.8_orig_2010-12/I/3554ea266fa9c72fb27980a45a0eded8.png)
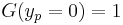 and decreases with
and decreases with 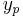 ; it is always positive. For
; it is always positive. For 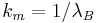 , we find
, we find
![P_\mathrm{Br} = {16 \over 3} \left[ Z_i^2 n_i n_e r_e^3 \right]
\left[ {c \over r_e} (m_e c^2 k_B T_e)^{1/2} \right] \alpha G(y_p)](/2010-wikipedia_en_wp1-0.8_orig_2010-12/I/46ab0ad327fc503393d91a8d33ab0039.png)
The first bracketed factor has units of 1/volume, while the second has units of power. Note the appearance of the fine structure constant 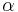 due to the quantum nature of
due to the quantum nature of 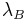 . In practical units, a commonly used version of this formula for
. In practical units, a commonly used version of this formula for  is [6]
is [6]
![P_\mathrm{Br} [\textrm{W/m}^3] = {Z_i^2 n_i n_e \over \left[7.69 \times 10^{18} \textrm{m}^{-3}\right]^2} T_e[\textrm{eV}]^{1/2}](/2010-wikipedia_en_wp1-0.8_orig_2010-12/I/3f967ab50e0102b982d1828b1cadc33c.png) .
.
This formula is 1.59 times the one given above, with the difference due to details of binary collisions. Such ambiguity is often expressed by introducing Gaunt factor  , e.g. in [7] one finds
, e.g. in [7] one finds
where everything is expressed in the CGS units.
Relativistic corrections
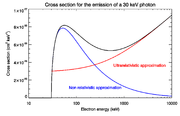
For very high temperatures there are relativistic corrections to this formula, that is, additional terms of the order of  [1]
[1]
Bremsstrahlung cooling
If the plasma is optically thin, the Bremsstrahlung radiation leaves the plasma, carrying part of the internal plasma energy. This effect is known as the Bremsstrahlung cooling. It is a type of radiative cooling. The energy carried away by Bremsstrahlung is called Bremsstrahlung losses and represent, respectively, a type of radiative losses. One generally uses the term Bremsstrahlung losses in the context when the plasma cooling is undesired, as e.g. in fusion plasmas.
Sources of Bremsstrahlung
X-ray tube
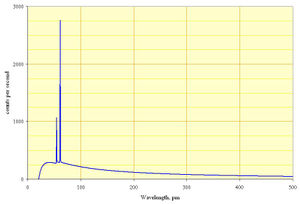
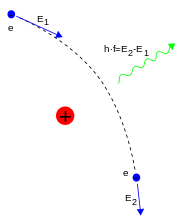
In an X-ray tube, electrons are accelerated in a vacuum by an electric field and shot into a piece of metal called the "target". X-rays are emitted as the electrons slow down (decelerate) in the metal. The output spectrum consists of a continuous spectrum of X-rays, with additional sharp peaks at certain energies (see graph on right). The continuous spectrum is due to bremsstrahlung, while the sharp peaks are characteristic X-rays associated with the atoms in the target. For this reason, bremsstrahlung in this context is also called continuous X-rays.[8]
The continuous spectrum has a sharp cutoff at low wavelength. This cutoff is due to the fixed energy of the incoming electrons. For example, if an electron in the tube is accelerated through 60 kV, then it will acquire a kinetic energy of 60 keV, and when it strikes the target it can create X-rays with energy of at most 60 keV, by conservation of energy. (This upper limit corresponds to the electron coming to a stop by emitting just one X-ray photon. Usually the electron emits many photons, and each has an energy less than 60 keV.) A photon with energy of at most 60 keV has wavelength of at least 21 pm, so the continuous X-ray spectrum has exactly that cutoff, as seen in the graph. More generally the formula for the low-wavelength cutoff is:[9]
 Å
Å
where h is Planck constant, c is the speed of light, V is the voltage that the electrons are accelerated through, e is the elementary charge, and Å is the angstrom unit of length (10−10 meters).
Beta decay
Beta particle-emitting substances sometimes exhibit a weak radiation with continuous spectrum that is due to Bremsstrahlung. In this context, Bremsstrahlung is a type of "secondary radiation", in that it is produced as a result of stopping (or slowing) the primary radiation (beta particles). In electron and positron emission the photon's energy comes from the electron/nucleon pair, with the spectrum of the bremsstrahlung decreasing continuously with increasing energy of the beta particle. In electron capture the energy comes at the expense of the neutrino, and the spectrum is greatest at about one third of the normal neutrino energy, reaching zero at zero energy and at normal neutrino energy.
Inner and outer Bremsstrahlung
The "inner" Bremsstrahlung arises from the creation of the electron and its loss of energy (due to the strong electric field in the region of the nucleus undergoing decay) as it leaves the nucleus. This is to be contrasted with the "outer" Bremsstrahlung due to the impingement on the nucleus of electrons coming from the outside (i.e., emitted by another nucleus).[10]
Radiation safety
In some cases, e.g. 32P, the Bremsstrahlung produced by shielding the beta radiation with the normally used dense materials (e.g. lead) is itself dangerous; in such cases, shielding must be accomplished with low density materials, e.g. Plexiglass (lucite), plastic, wood, or water;[11] because the rate of deceleration of the electron is slower, the radiation given off has a longer wavelength and is therefore less penetrating.
In astrophysics
The dominant luminous component in a cluster of galaxies is the 107 to 108 kelvin intracluster medium. The emission from the intracluster medium is characterized by thermal Bremsstrahlung. This radiation is in the energy range of X-rays and can be easily observed with space-based telescopes such as Chandra X-ray Observatory, XMM-Newton, ROSAT, ASCA, EXOSAT, Astro-E2, RHESSI and future missions like Con-X [2] and NeXT [3].
See also
- Cyclotron radiation
- Free electron laser
- Nuclear fusion: Bremsstrahlung losses
- Radiation length characterising energy loss by Bremsstrahlung by high energy electrons in matter
- Synchrotron light
- X-rays: History
|
|||||
References
- ↑ "Tesla's independent discovery of X rays, unlike Roentgen's, was primarily based upon sources that produced X rays by vacuum high field emission and the process now known as bremsstrahlung." See "Nikola Tesla Lecture Before the New York Academy of Sciences - April 6, 1897".
- ↑ Introduction to Electrodynamics, D. J. Griffiths, p.463-465
- ↑ A Plasma Formulary for Physics, Technology, and Astrophysics, D. Diver, p. 46-48.
- ↑ Radiation Processes in Plasmas, G. Bekefi
- ↑ Basic Principles of Plasmas Physics: A Statistical Approach, S. Ichimaru, p. 228.
- ↑ NRL Plasma Formulary, 2006 Revision, p. 58.
- ↑ Radiative Processes in Astrophysics, G.B. Rybicki & A.P. Lightman, p. 162.
- ↑ Electron microprobe analysis and scanning electron microscopy in geology, by S. J. B. Reed, 2005, page 12 Google books link
- ↑ Handbook of X-ray spectrometry by René Grieken, Andrzej Markowicz, page 3, Google books link
- ↑ Knipp, J.K.; G.E. Uhlenbeck (1936-06). "Emission of gamma radiation during the beta decay of nuclei". Physica 3 (6): 425–439. doi:10.1016/S0031-8914(36)80008-1. ISSN 0031-8914. http://www.sciencedirect.com/science/article/B6X42-4DS3862-34/2/5b2f20d7c387d34a07e7355bbcd9e39e. Retrieved 2010-05-12.
- ↑ http://www.oseh.umich.edu/TrainP32.pdf
Further reading
Eberhard Haug & Werner Nakel (2004). The elementary process of Bremsstrahlung. River Edge NJ: World Scientific. p. Scientific lecture notes in physics, vol. 73. ISBN 9812385789. http://books.google.com/books?hl=en&id=v4FMtIwTri8C&dq=bremsstrahlung+haug&printsec=frontcover&source=web&ots=THjay1eeFA&sig=aHe-xMFwT8jxhpAGJHDnxKC6Jjc#PPA29,M1.